Circular Motion Summary
Here’s an “ingelious” way on JC A Level H2 Physics Circular Motion summary
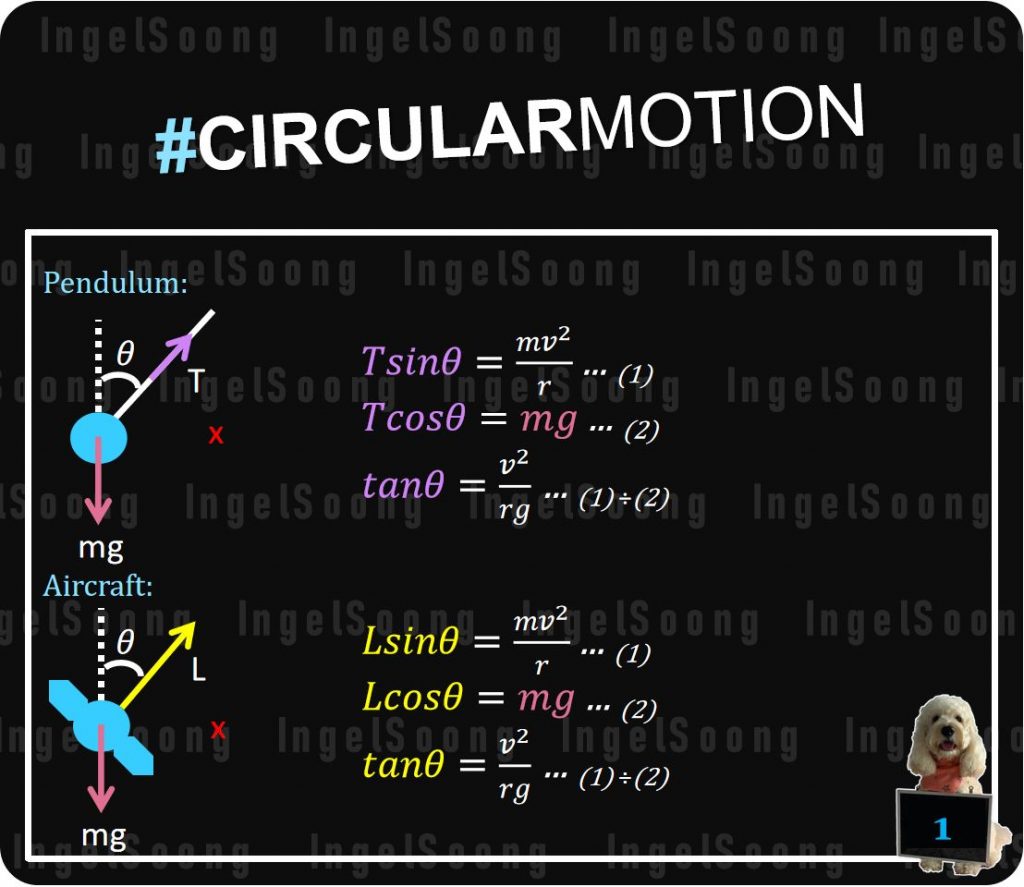
For pendulum:
T = tension on pendulum
mg = weight of pendulum
$Tsin\theta = \frac{mv^2}{r} … (1) $
$Tcos\theta = mg … (2) $
$tan\theta = \frac{v^2}{rg} …(1)\div(2) $
For aircraft:
L = lifting force on aircraft
mg = weight of aircraft
$Lsin\theta = \frac{mv^2}{r} … (1) $
$Lcos\theta = mg … (2) $
$tan\theta = \frac{v^2}{rg} …(1)\div(2)$
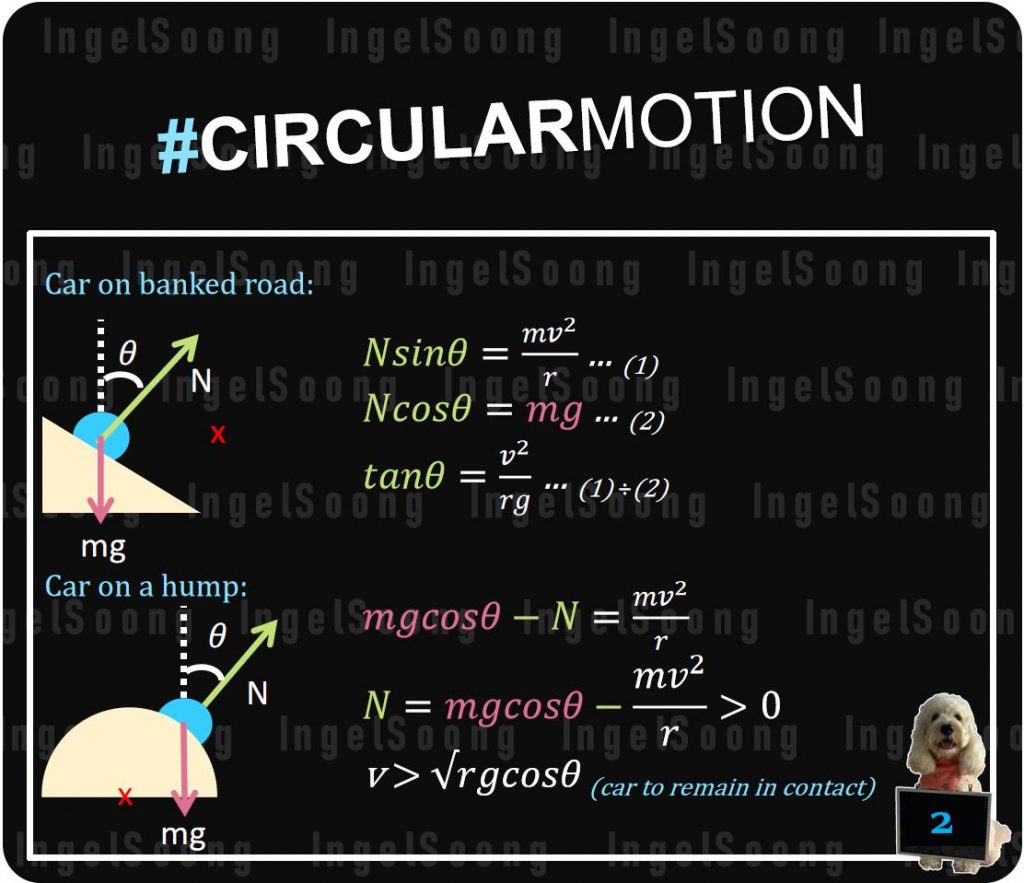
For car on banked road:
N = normal contact force on car
mg = weight of car
$Nsin\theta = \frac{mv^2}{r} … (1) $
$Ncos\theta = mg … (2) $
$tan\theta = \frac{v^2}{rg} … (1)\div(2)$
For car on hump:
N = normal contact force on car
mg = weight of car
$mgcos\theta – N = \frac{mv^2}{r} $
$N – mgcos\theta = \frac{mv^2}{r} > 0 $
$v > \sqrt{rgcos\theta} $ for car to remain in contact with road
For pendulum in vertical circular motion:
T = tension on pendulum
mg = weight of pendulum
$mgcos\theta + T = \frac{mv^2}{r} $
$T = \frac{mv^2}{r} – mgcos\theta $
$T = \frac{mv^2}{r} – mg $ at the top, when $\theta= 0^o$
$T = \frac{mv^2}{r} + mg $ at the top, when $\theta= 180^o$
$T = \frac{mv^2}{r} – mg > 0 $
$v > \sqrt{rgcos\theta} $ for string to be taut
For water in a pail in vertical circular motion:
T = tension on pendulum
mg = weight of pendulum
$mgcos\theta + N = \frac{mv^2}{r} $
$N = \frac{mv^2}{r} – mgcos\theta > 0 $
$v > \sqrt{rgcos\theta} $ for water to remain in pail