Gravitational Field Potential Gradient
Here’s an “ingelious” way to understand potential gradient F= − $ \frac{dU}{dr} $ related to gravitational field.
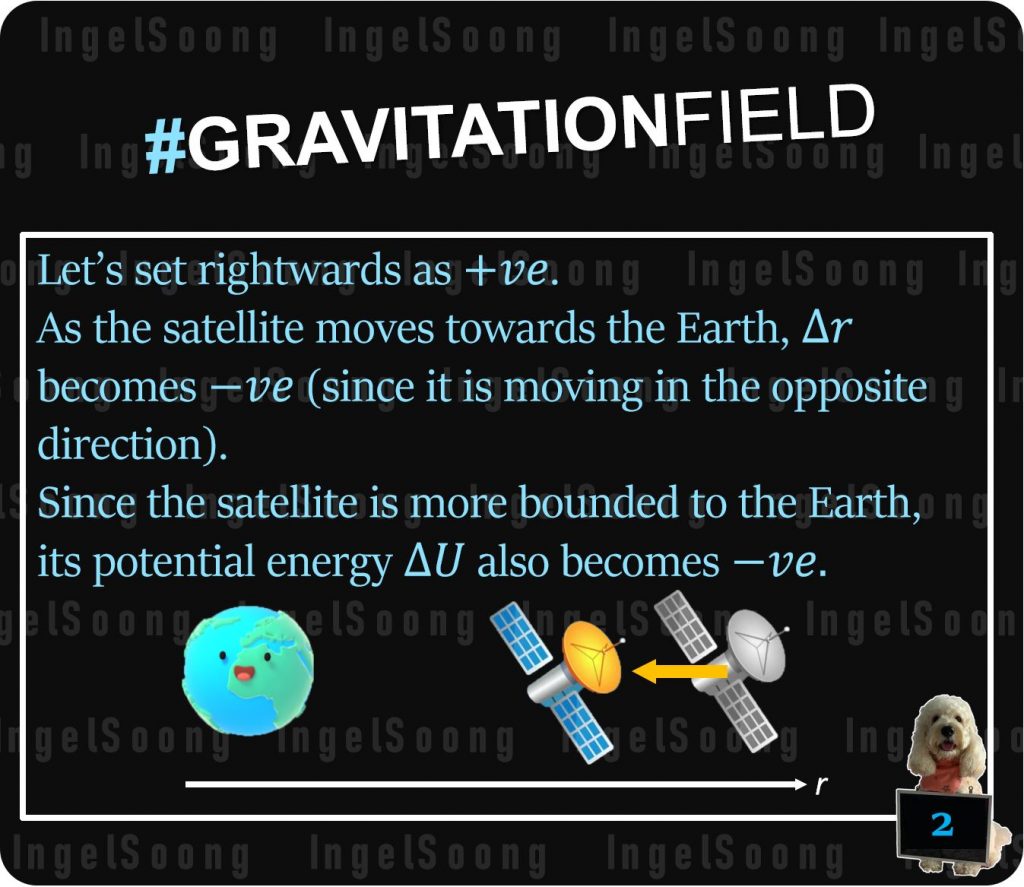
Consider a satellite moving towards the Earth.
Let’s set rightwards as +ve.
As the satellite moves towards the Earth, ∆r becomes −ve (since it is moving in the opposite direction).
Since the satellite is more bounded to the Earth, its potential energy $ \Delta U $ also becomes −ve
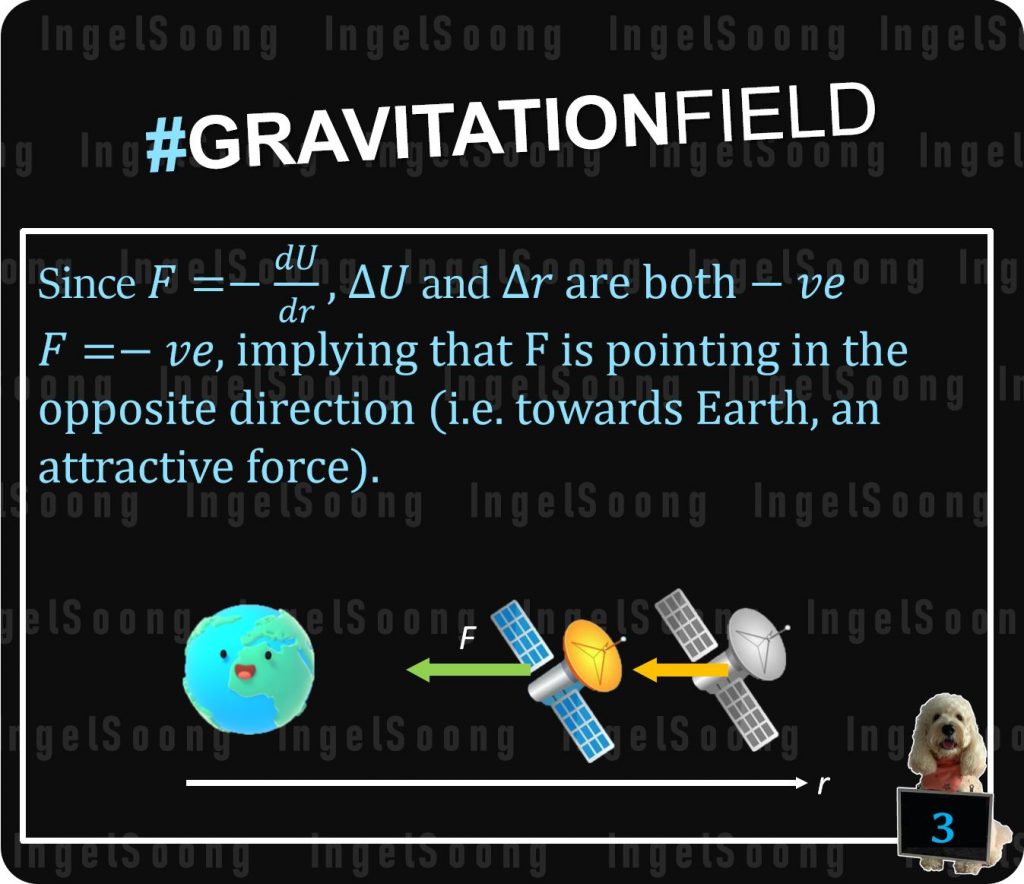
Since F = − $ \frac{dU}{dr} $, $ \Delta U$ and $ \Delta r$ are both −ve
F=−ve, implying that F is pointing in the opposite direction (i.e. towards Earth, an attractive force).
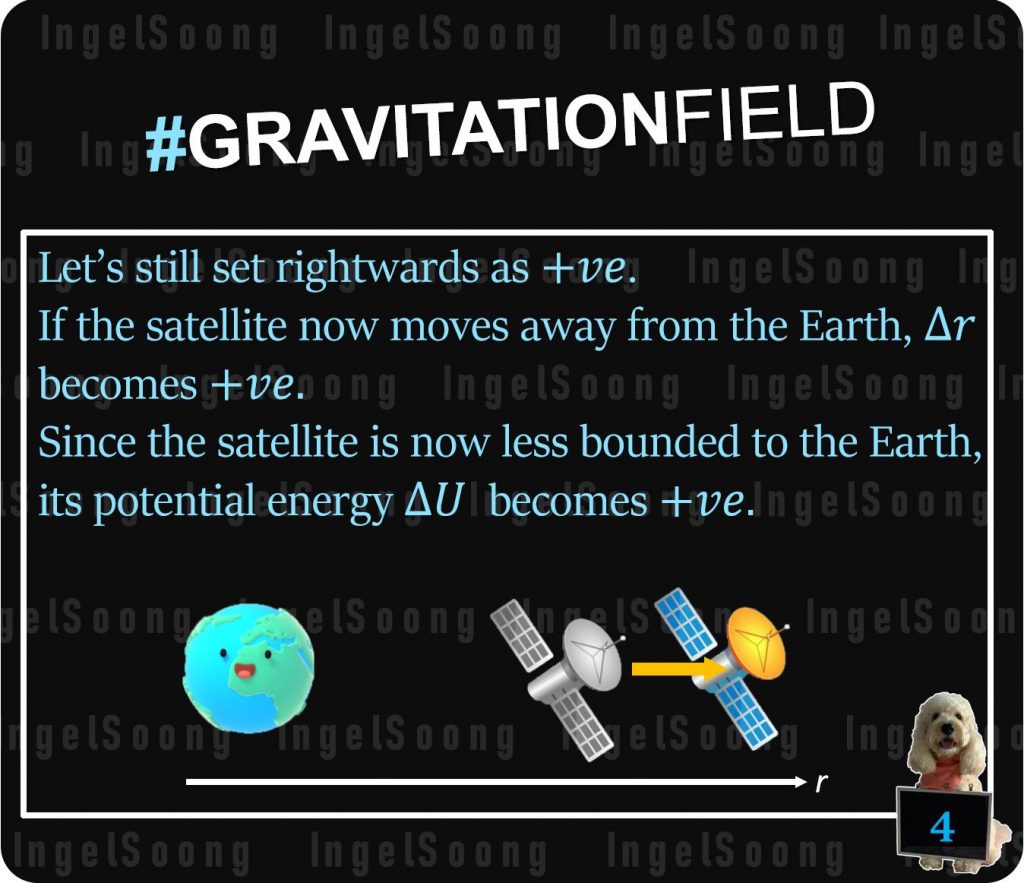
Let’s still set rightwards as +ve.
If the satellite now moves away from the Earth, ∆r becomes +ve.
Since the satellite is now less bounded to the Earth, its potential energy $ \delta U $ becomes +ve.
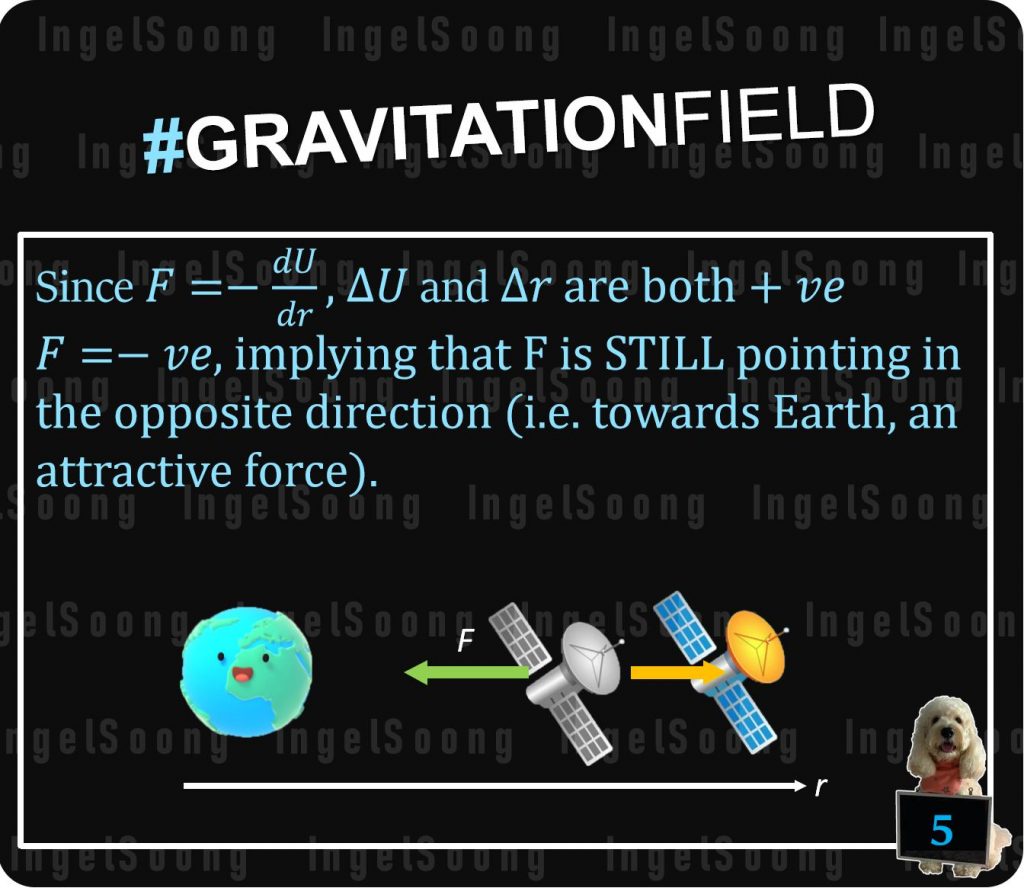
Since F= − $ \frac{dU}{dr} $, $ \Delta U $ and $ \Delta r $ are both +ve
F=−ve, implying that F is STILL pointing in the opposite direction (i.e. towards Earth, an attractive force).
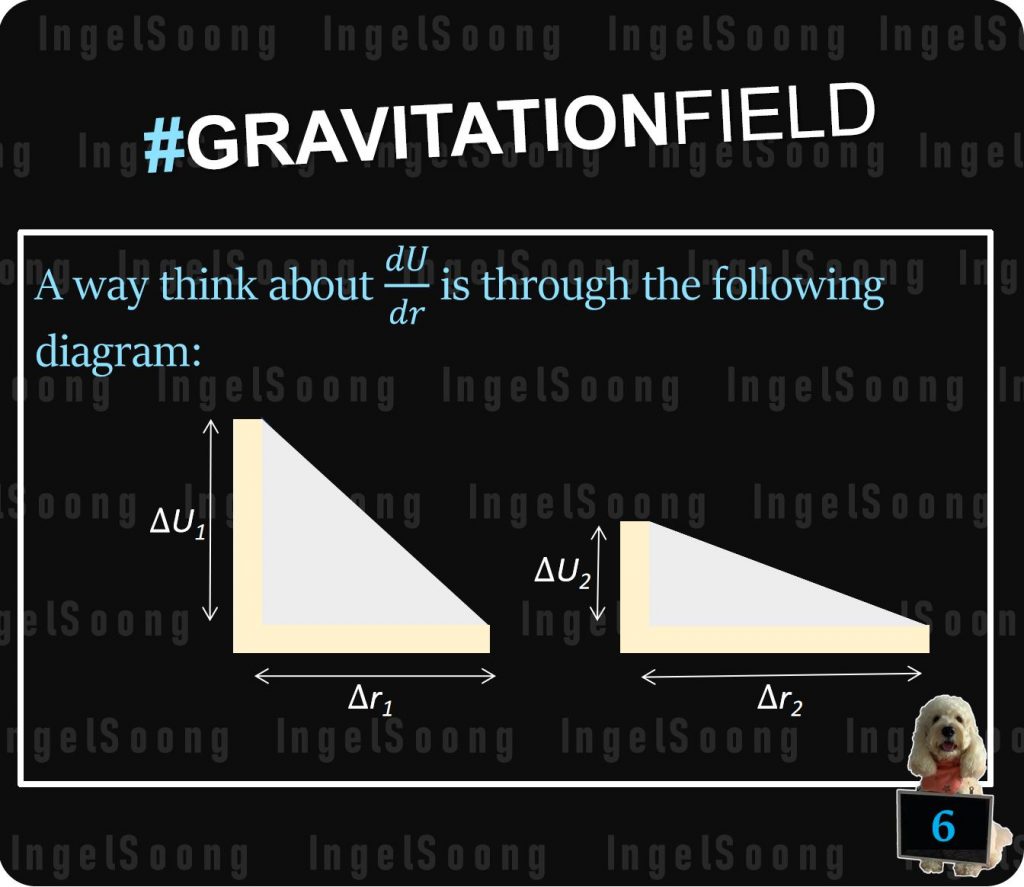
A way think about $ \frac{dU}{dr} $ is through the following diagram below (slide 6).
Since $ \Delta U_1 > \Delta U_2 $ and $\Delta r_1 < \Delta r_2 $, the potential gradient $F_1 > F_2 $ :
The force acts in the opposite direction of the potential gradient in line with the Conservation of Energy.
The larger the gradient, the larger the force is needed to add increasing amount of energy to the mass.