💯An Ingelious Way to Understand Kinematics Projectile Motion Questions

5/5 - 1 reviews

Kinematics Projectile Motion

Here’s an “ingelious” way to understand JC A Level H2 Physics projectile motion related to kinematics.
Let’s try to derive the optimum angle to throw a javelin for maximum range, assuming no air resistance:

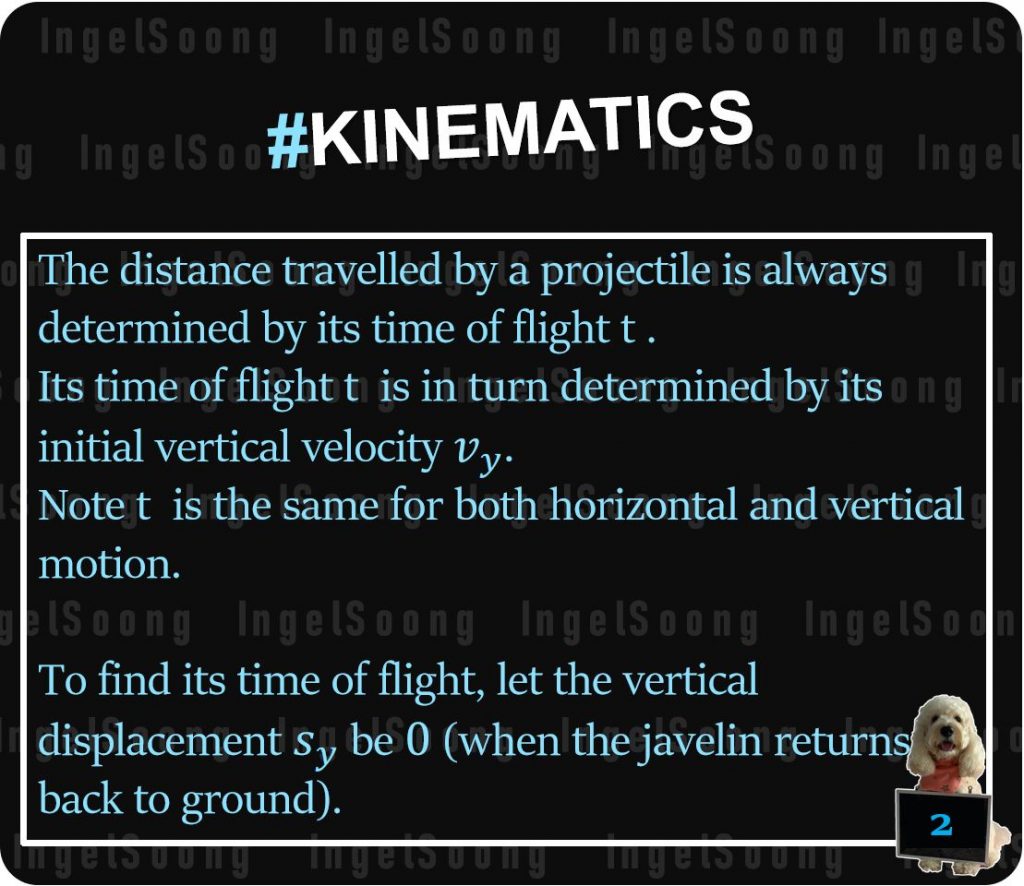
The distance travelled by a projectile is always determined by its time of flight $t$ .
Its time of flight $t$ is in turn determined by its initial vertical velocity $v_y$ .
Note $t$ is the same for both horizontal and vertical motion.
To find its time of flight, let the vertical displacement $s_y$ be 0 (when the javelin returns back to ground)
Set upwards as positive,
$s_y = u_yt + \frac{1}{2} a_yt^2$

It is good practice not to change the sign of the formula, but use $(-g)$
$0 = usin \theta t + \frac{1}{2} (-g)t^2$
$0 = usin \theta t - \frac{1}{2} gt^2$
$0 = (usin \theta - \frac{1}{2} gt)(t)$
$t = 0 \, (reject) \,$ or $\, t = \frac{2usin\theta}{g}$